The Standard Model (SM) of particle physics is the most successful theory of all time. However, we know that the SM is incomplete at some levels, which is due in part to the presence of large neutrinos. Neutrinos are fast-moving SM fermions (leptons) that only have weak gravitational interactions with other particles. Neutrinos are omnipresent; hundreds of thousands of them from the beginning of the cosmos are currently traveling through your body! However, the SM predicts that neutrinos should have no mass, which is inconvenient because their masses have been measured to be non-zero by particle physicists. As a result, neutrinos have been added to the growing number of elephants (such as dark matter and dark energy) in the beyond-SM room.
Particle physicists are attempting to put an upper bound on neutrino masses to complement the present lower bound derived from neutrino oscillations. The current best constraint from the KATRIN experiment is 0.8 eV (for comparison, the electron mass is 511 thousand eV), however it turns out that cosmology can constrain the sum of neutrino masses even better! This sum is already constrained to be less than 0.12 eV by the Planck Cosmic Microwave Background (CMB) and Baryon Acoustic Oscillation (BAO) data, and future datasets are projected to bring this upper bound even lower.
This all sounds fantastic, but the news in today’s paper puts a damper on the festivities (in a good way). Existing cosmic restrictions often assume a certain choice of neutrino physics and the Lambda Cold Dark Matter (CDM) model (as in the Planck+BAO example). But what if we offer a little more latitude in terms of the types of neutrino physics we allow? How can we investigate these options? Today’s article answers that question – specifically, it investigates how much wiggle space the CMB constraints leave on neutrino mass when moving beyond the “conventional” CDM cosmological model.
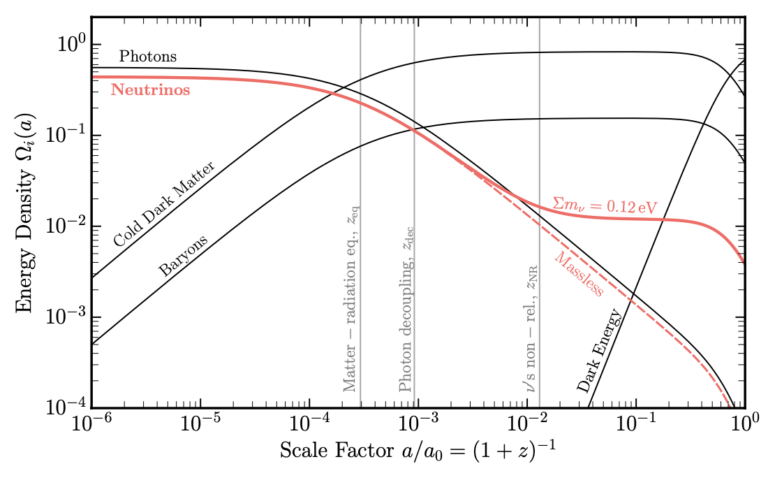
Figure 1: Energy density progression of cosmological species over time (at the level of background dynamics). A scale value of 1 represents today, whereas 0.001 represents the time of photon decoupling linked with the CMB. The red curve shows how, depending on whether neutrinos are heavy or not, the time evolution of neutrino energy density bifurcates at late times. Today’s paper’s figure 1.
In the early and late universes, neutrinos behave differently (Figure 1). In the first, neutrinos behave like radiation and are described by their relativistic energy density R (the solid red curve resembles that of cold dark matter), whereas in the second, they behave like matter and are described by their non-relativistic energy density NR (the red curve resembles that of photons). Neutrinos undergo a transition from relativistic to nonrelativistic in the middle, which we are unable to see well (splitting of red solid and dashed curves in Fig. 1). The CMB data can constrain neutrinos’ behavior around decoupling (R) through characteristic scales corresponding to baryon oscillations, as well as later on through neutrinos’ contribution to the universe’s background expansion (NR). When the neutrinos are non-relativistic, the BAO can help constrain NR by probing low redshifts.
Makes neutri-no difference to me
Today’s authors consider several choices of neutrino physics models (parameterized through their phase space distribution functions) that are distinct from the standard ΛCDM choice of particles obeying Fermi-Dirac statistics. These models, for example, correspond to especially high or low energy neutrino populations that may or may not have “dark radiation” mixed into the model. For each model, the authors perform posterior inference of massive neutrino parameters using the Planck Cosmic Microwave Background (CMB) anisotropy power spectrum temperature and polarization data. This high-redshfit CMB data is then combined with low-redshift Baryon Acoustic Oscillation (BAO) data from BOSS.
To me, it makes no difference
The authors of today’s paper look at a variety of neutrino physics models (parameterized by their phase space distribution functions) that are different from the typical CDM option of Fermi-Dirac statistics obeying particles. These models, for example, correlate to neutrino populations with unusually high or low energies, and may or may not include “black radiation.” The authors use the Planck Cosmic Microwave Background (CMB) anisotropy power spectrum temperature and polarization data to undertake posterior inference of large neutrino parameters for each hypothesis. The low-redshift Baryon Acoustic Oscillation (BAO) data from BOSS is then merged with the high-redshift CMB data.
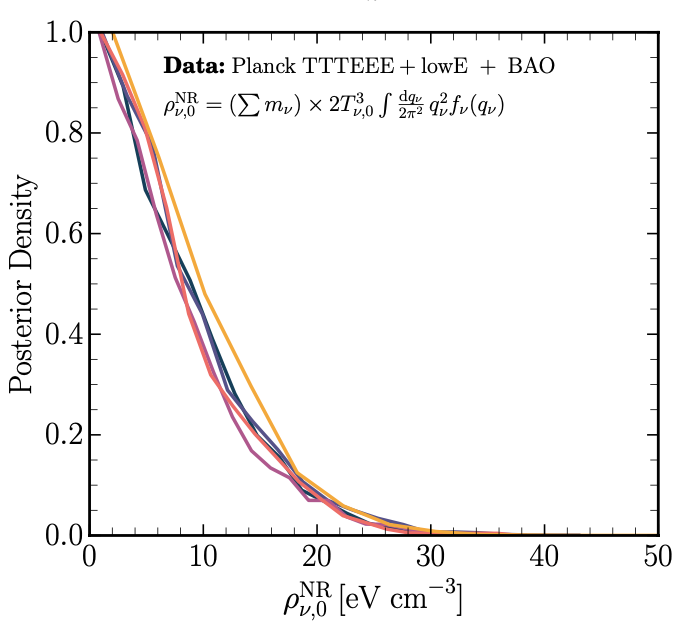
Figure 2: Planck+BOSS Baryon Acoustic Oscillation data produced posterior limits on the non-relativistic massive neutrino density (as observed today). Different colored curves correspond to very different choices of massive neutrino physics (CDM in dark blue), but they are all restricted at around the same level, indicating that the data cannot discriminate between these models. Extracted from today’s paper’s Figure 2.
Figure 2 shows the findings of this research for the non-relativistic energy density (different model choices are the different colored curves). The precise interpretation of non-relativistic energy is not particularly important here, but it is clear that all of the modeling options produce a similar constraint to the CDM constraint (dark blue curve), indicating that this data cannot distinguish between the various neutrino physics choices encoded in the different curves. Should we really be so sure of our “mainstream” CDM model for neutrinos after learning this lesson?
The CMB and the BAO both have opinions on neutrino mass
Today’s authors construct a “generalized” large neutrino physics model to go beyond the standard (CDM) assumptions of neutrino physics. This model is flexible enough to account for neutrino masses that correspond to the prior selections in Fig. 2 that were bound separately. The authors hope to establish a model-independent constraint on the sum of the neutrino masses, as well as the other features to which the CMB+BAO data is sensitive, by employing the more flexible model. The posterior constraints on the three neutrino parameters in this model are shown in Figure 3.
Figure 3: Using Planck+BAO data and the “generalized” variant of the massive neutrino model, posterior restrictions on a subset of the parameters are imposed. At early times, Neff measures the (relativistic) energy density of neutrinos, whereas NR,0 measures the (non-relativistic) energy density of neutrinos. Within the range of acceptable values, the neutrino mass is loosely limited. Figure 4 in today’s issue of the publication.
We still have the non-relativistic energy density NR, but instead of many fixed neutrino physics models and neutrino masses, we now have the parameter Neff and the sum of neutrino masses itself (as in Fig. 2). The relativistic energy density R is intimately related to the parameter Neff, which essentially re-expresses R in terms of the photon energy density. The authors want you to remember that when neutrino physics modeling is loosened, not only does the CMB+BAO constraint on the sum of neutrino masses relax dramatically, but the constraint on Neff is not significantly worse than the CDM constraint.
More evidence a-massing
According to the findings of today’s paper, the sum of neutrino masses in the general neutrino physics case has greater wiggle space than in the CDM case. The authors demonstrated that, at least in terms of Planck CMB and BOSS BAO data, the subtleties of neutrino physics will remain concealed for the time being. But don’t worry, large-scale structure is on the way! As a last thought, the authors of today’s paper propose that future weak lensing or galaxy clustering analysis may be able to tighten neutrino mass limits even for the extended model under consideration. Keep an eye out for *nu* physics on the horizon!